This post continues Part 3 of a series on the Kelly criterion and its application to sports betting. Part 1 provides an introduction to the Kelly criterion and a worked example. Part 2 provides a simple derivation. This post discusses fractional Kelly betting, where a fraction of the amount recommended by the Kelly criterion is placed on each bet.

Why consider fractional Kelly betting?

There are two popular criticisms of the Kelly criterion. The first concerns Kelly betting in general, and the second concerns the application of the Kelly criterion to sports betting.

The first criticism is the roller-coaster ride that your account balance takes when you implement a Kelly strategy. The Kelly criterion can provide optimal bet amounts that exceed 50% of the account balance, which creates a high degree of volatility.

The second criticism is that, when applied to sports betting, the Kelly criterion does not account for the uncertainty in your perceived probability of occurrence. Kelly betting can be applied to Blackjack with a high degree of certainty in the calculated probabilities. With sports betting, however, you may feel the probability of an outcome is 50%, but you do not know that with certainty. The true probability may lie between 40% and 60%, or even 20% and 80%. Applying the Kelly criterion without acknowledging the uncertainty in the probability can lead to ruin.

These criticisms have led many practitioners to adopt fractional Kelly betting, also known as partial Kelly betting. It involves taking the bet amount recommended by the Kelly criterion and multiplying it by a certain fraction. This results in less volatile returns and a lower chance of the account balance hitting zero.

The Kelly criterion formula revisited

The Kelly criterion calculates the fraction, f, of the account balance that should be placed on a bet, given the available odds and your perceived probability of winning. The formula depends on how you express the betting odds, so two versions are presented below. Version A uses the decimal odds system that is popular in Australia. Decimal odds of 2.50 mean that if you win, a $10 bet would result in a $25 payout and a $15 profit. Version B uses the “b to 1″ odds system, where odds of “3 to 1″ mean that if you win, a $10 bet would result in a payout of $40 and a profit of $30.

Version:		A		B
Formula: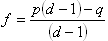
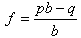Variables:		f = fraction of your bankroll to bet d = decimal betting odds p = probability of bet winning q = 1 – p = probability of bet losing		f = fraction of your bankroll to bet b = “b to 1″ betting odds p = probability of bet winning q = 1 – p = probability of bet losing

Fractional Kelly betting

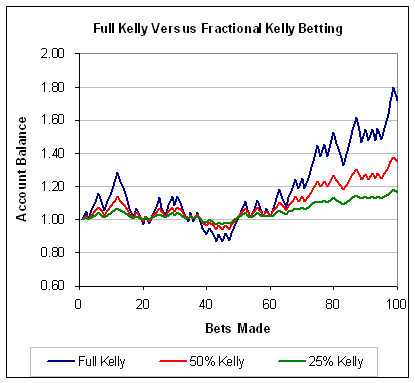
With fractional Kelly betting, you multiply f by a number between 0 and 1. Hence, if we use h as our multiplier, where 0 < h < 1, we now bet h*f of our account balance instead of f. The lower the value of h, the more conservative the strategy, because you are betting a smaller percentage of your account balance. For example, a quarter Kelly strategy, where h = 1/4, is more conservative than a half Kelly strategy, where h = 1/2. The graph below provides a stylized comparison of a full Kelly versus a half Kelly and a quarter Kelly. The graph has been drawn by simulating 100 bets with odds of 2.10 and a perceived probability of occurrence (which is modeled as being true) of 50%. Observe that the full Kelly criterion results in the most volatility, but is also expected to provide the highest long-term growth.

How to implement fractional Kelly betting

With full Kelly betting, the only ambiguous variable is your perceived probability, p, of an outcome occurring. The betting odds are known with certainty, and q is simply 1 – p. With fractional Kelly betting you now have the additional decision of how much to reduce f by. An aggressive strategy is to multiply f by a number close to 1, and a conservative strategy is to multiply f by a number close to zero.

So how do you choose a value for h?

A non-technical approach is to set an arbitrary value for h, say h = 0.25, and commence Kelly betting. If after a few hundred bets your strategy has enjoyed positive results, you could increase h to 0.30 for the next set of bets, and proceed in this manner until you find a value for h that you feel comfortable with.

A more technical approach is to record for a few hundred bets over time:

1. The betting odds
2. Your perceive probability of occurrence
3. The Kelly criterion value for f
4. The result of that bet (win/lose)

For an arbitrary value of h, simulate your portfolio performance had you bet h*f on these fixtures. Excel solver can be used to find the value of h that would have maximised your account balance growth over time. Alternatively, you could calculate the value of h that, in retrospect, would have provided a level of volatility that you feel comfortable with. This value of h could then be applied, and tweaked over time, as more betting data are recorded.

Variable fractional Kelly betting

While some practitioners use the same value of h for all bets, one alternative is to vary h depending on your confidence in the chosen value of p. Recall that the Kelly criterion calculates the optimal bet amount based on the disparity between p and the probability that is implied by the betting odds (1/d). By applying variable fractional Kelly betting, your bet amount will depend both on the disparity between p and 1/d, and your confidence in the chosen value for p.

Let h equal the maximum fraction you would ever consider to multiply f by. If you set h = 1, then in some circumstances you are willing to implement full Kelly betting. Alternatively you may only wish to bet at most 0.5*f, hence h = 0.5.

Now let the variable g represent your confidence in the chosen value of p, where 0 < g < 1. g = 0 equates to no confidence, and g = 1 represents full confidence. For each bet, calculate f and g, and bet the fraction f*h*g of your account balance. If you are absolutely certain in p, then g = 1, and you would bet your maximum fraction h*f.

The weakness in this approach is it provides no guidance on how to set g. How do you measure the level of certainty in your perceived probability? You may be more confident in your knowledge of some sports than others, but how to you quantify that? If you use a betting tracker spreadsheet you could compare your historical performance across different sports. Stronger performance indicates that you are better able to assess the probabilities in that sport. One approach is to set g based on your performance percentile for that sport compared to the rest. For example, if you regularly bet on eleven sporting markets, then you could set g as follows.

Sport	Return	Rank	g
Super Rugby	3%	7	0.4
Test Match Cricket	2%	8	0.3
Premier League Football	8%	3	0.8
Men’s Tennis	6%	4	0.7
NBA Basketball	5%	5	0.6
Women’s Tennis	-3%	10	0.1
Formula 1	-4%	11	0.0
NCAA Basketball	-1%	9	0.2
NRL Line Betting	12%	1	1.0
NRL Head to Head	11%	2	0.9
AFL Line Betting	4%	6	0.4

g has been calculated above as (11 – rank)/(11 – 1). Hence, for h = 0.5, you would bet 0.4*f on Premier League fixtures and 0.3*f on NBA fixtures. The value of h could be chosen using the methods outlined in the previous section.